Number Theory #

Syllabus #

File: math102-syllabus.pdf

Notes during orientation #

• No complex numbers in this class
• If we use the variable \( n \) , it is assumed it is an integer
• \( p \) assumes a prime number

Warm up #

Breakdown according to oldest age:

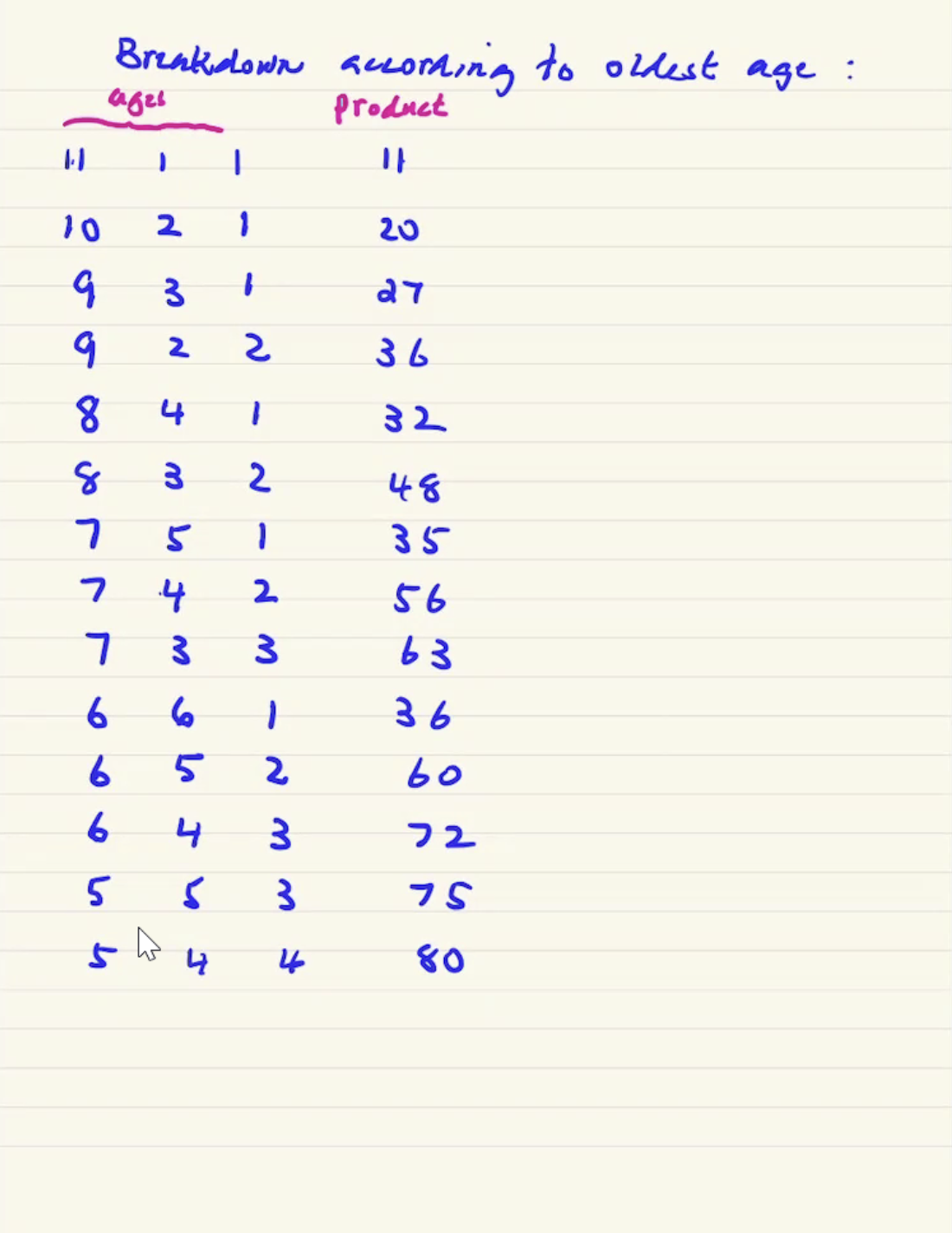
• note that 36 appears twice, since Mary needs more time to figure it out it must be this value
• When Rafael talks about his “eldest” son that means that one son is older than the rest, so the answer is 9 2 2

Important sets #

• \( \mathbb{Z} = \{\ldots, -2, -1, 0, 1, 2, 3, \ldots\} \) , the set of all integers.
• \( \mathbb{Z}^+ = \{1, 2, 3, \ldots\} \) , the set of all positive integers.
• \( \mathbb{N} = \{0, 1, 2, 3, \ldots\} \)
• \( \mathbb{Q} = \) all numbers that can be written as \( \frac{a}{b} \) where \( a \) and \( b \) \( \in \mathbb{Z} \) . So, \( \mathbb{Z} \) is contained in \( \mathbb{Q} \) , but \( \mathbb{Q} \) is not contained in \( \mathbb{Z} \) .
• \( \mathbb{R} \) is the set of all real numbers, also denoted by \( (\infty, \infty) \) . \( \mathbb{Q} \) is contained in \( \mathbb{R} \) , but \( \mathbb{R} \) has elements that are not in \( \mathbb{Q} \) .

Primes and composites #

Any integer \( > 1 \) which is only divisible by \( 1 \) and itself is called a prime number.

Any integer \( > 1 \) , which is not a prime, is a composite number.

Definition: The Fundamental Theorem of Arithmetic

1. All composite numbers are products of primes. We call these products prime factorizations.
2. Each composite number has only one prime factorization (sometimes abbreviated as pf), up to order.

Finding the prime factorization of composite numbers #

Divide by the smallest prime which goes in repeatedly. So,